home | what's new | other sites | contact | about

Word Gems 

exploring self-realization, sacred personhood, and full humanity

Quantum Mechanics

return to "Quantum Mechanics" main-page

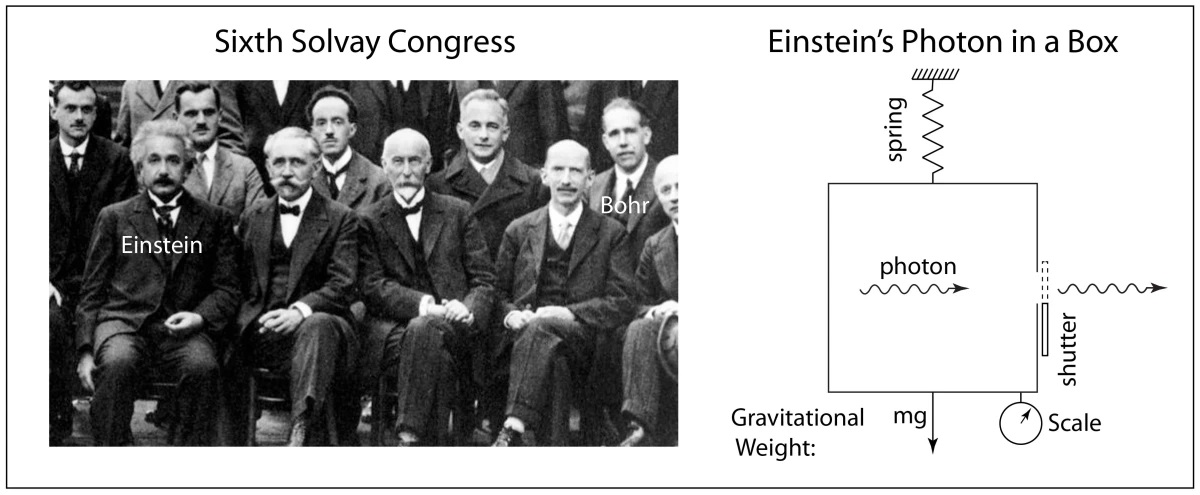
The following is from David D. Nolte: The Solvay Debates: Einstein versus Bohr

Einstein is the alpha of the quantum. Einstein is also the omega. Although he was the one who established the quantum of energy and matter (see my Blog Einstein vs Planck), Einstein pitted himself in a running debate against Niels Bohr’s emerging interpretation of quantum physics that had, in Einstein’s opinion, severe deficiencies.

Between sessions during a series of conferences known as the Solvay Congresses over a period of eight years from 1927 to 1935, Einstein constructed challenges of increasing sophistication to confront Bohr and his quasi-voodoo attitudes about wave-function collapse.

To meet the challenge, Bohr sharpened his arguments and bested Einstein, who ultimately withdrew from the field of battle. Einstein, as quantum physics’ harshest critic, played a pivotal role, almost against his will, establishing the Copenhagen interpretation of quantum physics that rules to this day, and also inventing the principle of entanglement which lies at the core of almost all quantum information technology today.

Editor’s note: Notice how this process of determining truth works. Debate is a crucible in which unclear concepts are brought into sharper clarity. Today totalitarians, in their Orwellian heavy-handedness, quickly move to censorship, to self-serving charges of “misinformation”, when their position is questioned. But consider the arrogance. Who is to determine what is “misinformation” and what is truth? – certainly not an elitist cabal of hoodwinkers and charlatans, but only those who allow for the purging fires of rigorous and honest public debate.

Debate Timeline

Fifth Solvay Congress: 1927 October Brussels: Debate Round 1. Einstein and ensembles

Sixth Solvay Congress: 1930 Debate Round 2. Photon in a box

Seventh Solvay Congress: 1933. Einstein absent (visiting the US when Hitler takes power, decides not to return to Germany.)

Physical Review 1935: Debate Round 3. EPR paper and Bohr’s response. Schrödinger’s Cat
   

The Solvay congresses were unparalleled scientific meetings of their day. They were attended by invitation only, and invitations were offered only to the top physicists concerned with the selected topic of each meeting.  The Solvay congresses were held about every three years always in Belgium, supported by the Belgian chemical industrialist Ernest Solvay.  The first meeting, held in 1911, was on the topic of radiation and quanta.

The fifth meeting, held in 1927, was on electrons and photons and focused on the recent rapid advances in quantum theory. The old quantum guard was invited—Planck, Bohr and Einstein. The new quantum guard was invited as well—Heisenberg, de Broglie, Schrödinger, Born, Pauli, and Dirac. 

Heisenberg and Bohr joined forces to present a united front meant to solidify what later became known as the Copenhagen interpretation of quantum physics. 

The basic principles of the interpretation include the wavefunction of Schrödinger, the probabilistic interpretation of Born, the uncertainty principle of Heisenberg, the complementarity principle of Bohr and the collapse of the wavefunction during measurement. 

The chief conclusion that Heisenberg and Bohr sought to impress on the assembled attendees was that the theory of quantum processes was complete, meaning that unknown or uncertain characteristics of measurements could not be attributed to lack of knowledge or understanding, but were fundamental and permanently inaccessible.

Einstein was not convinced with that argument, and he rose to his feet to object after Bohr’s informal presentation of his complementarity principle. Einstein insisted that uncertainties in measurement were not fundamental, but were caused by incomplete information, that , if known, would accurately account for the measurement results. 

Bohr was not prepared for Einstein’s critique and brushed it off, but what ensued in the dining hall and the hallways of the Hotel Metropole in Brussels over the next several days has become one of the most famous scientific debates of the modern era, known as the Bohr-Einstein debate on the meaning of quantum theory. 

The debate gently raged night and day through the fifth congress, and was renewed three years later at the 1930 congress. It finished, in a final flurry of published papers in 1935 that launched some of the central concepts of quantum theory, including the idea of quantum entanglement and, of course, Schrödinger’s cat.

Einstein’s strategy, to refute Bohr, was to construct careful thought experiments that envisioned perfect experiments, without errors, that measured properties of ideal quantum systems. His aim was to paint Bohr into a corner from which he could not escape, caught by what Einstein assumed was the inconsistency of complementarity. 

Einstein’s “thought experiments” used electrons passing through slits, diffracting as required by Schrödinger’s theory, but being detected by classical measurements. Einstein would present a thought experiment to Bohr, who would then retreat to consider the way around Einstein’s arguments, returning the next hour or the next day with his answer, only to be confronted by yet another clever device of Einstein’s clever imagination that would force Bohr to retreat again. 

The spirit of this back and forth encounter between Bohr and Einstein is caught dramatically in the words of Paul Ehrenfest who witnessed the debate first hand, partially mediating between Bohr and Einstein, both of whom he respected deeply.

“Brussels-Solvay was fine!… BOHR towering over everybody. At first not understood at all …, then step by step defeating everybody.  Naturally, once again the awful Bohr incantation terminology.  Impossible for anyone else to summarise … (Every night at 1 a.m., Bohr came into my room just to say ONE SINGLE WORD to me, until three a.m.) It was delightful for me to be present during the conversation between Bohr and Einstein. Like a game of chess, Einstein all the time with new examples. In a certain sense a sort of Perpetuum Mobile of the second kind to break the UNCERTAINTY RELATION. Bohr from out of philosophical smoke clouds constantly searching for the tools to crush one example after the other. Einstein like a jack-in-the-box; jumping out fresh every morning. Oh, that was priceless. But I am almost without reservation pro Bohr and contra Einstein. His attitude to Bohr is now exactly like the attitude of the defenders of absolute simultaneity towards him …” [1]

The most difficult example that Einstein constructed during the fifth Solvary Congress involved an electron double-slit apparatus that could measure, in principle, the momentum imparted to the slit by the passing electron, as shown in Fig.3. The electron gun is a point source that emits the electrons in a range of angles that illuminates the two slits. The slits are small relative to a de Broglie wavelength, so the electron wavefunctions diffract according to Schrödinger’s wave mechanics to illuminate the detection plate. Because of the interference of the electron waves from the two slits, electrons are detected clustered in intense fringes separated by dark fringes.

So far, everyone was in agreement with these suggested results. The key next step is the assumption that the electron gun emits only a single electron at a time, so that only one electron is present in the system at any given time. Furthermore, the screen with the double slit is suspended on a spring, and the position of the screen is measured with complete accuracy by a displacement meter. When the single electron passes through the entire system, it imparts a momentum kick to the screen, which is measured by the meter. It is also detected at a specific location on the detection plate. Knowing the position of the electron detection, and the momentum kick to the screen, provides information about which slit the electron passed through, and gives simultaneous position and momentum values to the electron that have no uncertainty, apparently rebutting the uncertainty principle.            
 

Fig. 3 Einstein’s single-electron thought experiment in which the recoil of the screen holding the slits can be measured to tell which way the electron went. Bohr showed that the more “which way” information is obtained, the more washed-out the interference pattern becomes.

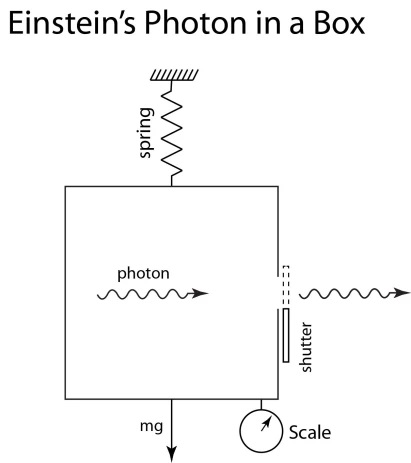
This challenge by Einstein was the culmination of successively more sophisticated examples that he had to pose to combat Bohr, and Bohr was not going to let it pass unanswered. With ingenious insight, Bohr recognized that the key element in the apparatus was the fact that the screen with the slits must have finite mass if the momentum kick by the electron were to produce a measurable displacement. But if the screen has finite mass, and hence a finite momentum kick from the electron, then there must be an uncertainty in the position of the slits. 

This uncertainty immediately translates into a washout of the interference fringes. In fact the more information that is obtained about which slit the electron passed through, the more the interference is washed out. 

It was a perfect example of Bohr’s own complementarity principle. The more the apparatus measures particle properties, the less it measures wave properties, and vice versa, in a perfect balance between waves and particles.

Einstein grudgingly admitted defeat at the end of the first round, but he was not defeated. Three years later he came back armed with more clever thought experiments, ready for the second round in the debate.

At the Solvay Congress of 1930, Einstein was ready with even more difficult challenges. His ultimate idea was to construct a box containing photons, just like the original black bodies that launched Planck’s quantum hypothesis thirty years before. The box is attached to a weighing scale so that the weight of the box plus the photons inside can be measured with arbitrarily accuracy. A shutter over a hole in the box is opened for a time T, and a photon is emitted. Because the photon has energy, it has an equivalent weight (Einstein’s own famous E = mc2), and the mass of the box changes by an amount equal to the photon energy divided by the speed of light squared: m = E/c2. If the scale has arbitrary accuracy, then the energy of the photon has no uncertainty. In addition, because the shutter was open for only a time T, the time of emission similarly has no uncertainty. Therefore, the product of the energy uncertainty and the time uncertainty is much smaller than Planck’s constant, apparently violating Heisenberg’s precious uncertainty principle.

Bohr was stopped in his tracks with this challenge. Although he sensed immediately that Einstein had missed something (because Bohr had complete confidence in the uncertainty principle), he could not put his finger immediately on what it was. That evening he wandered from one attendee to another, very unhappy, trying to persuade them and saying that Einstein could not be right because it would be the end of physics.  At the end of the evening, Bohr was no closer to a solution, and Einstein was looking smug. However, by the next morning Bohr reappeared tired but in high spirits, and he delivered a master stroke. Where Einstein had used special relativity against Bohr, Bohr now used Einstein’s own general relativity against him.

The key insight was that the weight of the box must be measured, and the process of measurement was just as important as the quantum process being measured—this was one of the cornerstones of the Copenhagen interpretation. 

So Bohr envisioned a measuring apparatus composed of a spring and a scale with the box suspended in gravity from the spring. As the photon leaves the box, the weight of the box changes, and so does the deflection of the spring, changing the height of the box. This change in height, in a gravitational potential, causes the timing of the shutter to change according to the law of gravitational time dilation in general relativity. By calculating the the general relativistic uncertainty in the time, coupled with the special relativistic uncertainty in the weight of the box, produced a product that was at least as big as Planck’s constant—Heisenberg’s uncertainty principle was saved!

Fig. 4 Einstein’s thought experiment that uses special relativity to refute quantum mechanics. Bohr then invoked Einstein’s own general relativity to refute him.

Entanglement and Schrödinger’s Cat

Einstein ceded the point to Bohr but was not convinced. He still believed that quantum mechanics was not a “complete” theory of quantum physics and he continued to search for the perfect thought experiment that Bohr could not escape. Even today when we have become so familiar with quantum phenomena, the Copenhagen interpretation of quantum mechanics has weird consequences that seem to defy common sense, so it is understandable that Einstein had his reservations.

After the sixth Solvay congress Einstein and Schrödinger exchanged many letters complaining to each other about Bohr’s increasing strangle-hold on the interpretation of quantum mechanics. Egging each other on, they both constructed their own final assault on Bohr. The irony is that the concepts they devised to throw down quantum mechanics have today become cornerstones of the theory.

For Einstein, his final salvo was “Entanglement”. For Schrödinger, his final salvo was his “cat”. Today, Entanglement and Schrödinger’s Cat have become enshrined on the altar of quantum interpretation even though their original function was to thwart that interpretation.

The final round of the debate was carried out, not at a Solvay congress, but in the Physical Review journal by Einstein [2] and Bohr [3], and in the Naturwissenshaften by Schrödinger [4].

In 1969, Heisenberg looked back on these years and said,

"To those of us who participated in the development of atomic theory, the five years following the Solvay Conference in Brussels in 1927 looked so wonderful that we often spoke of them as the golden age of atomic physics. The great obstacles that had occupied all our efforts in the preceding years had been cleared out of the way, the gate to an entirely new field, the quantum mechanics of the atomic shells stood wide open, and fresh fruits seemed ready for the picking." [5]

References

[1] A. Whitaker, Einstein, Bohr, and the quantum dilemma : from quantum theory to quantum information, 2nd ed. Cambridge University Press, 2006. (pg. 210)

[2] A. Einstein, B. Podolsky, and N. Rosen, “Can quantum-mechanical description of physical reality be considered complete?,” Physical Review, vol. 47, no. 10, pp. 0777-0780, May (1935)

[3] N. Bohr, “Can quantum-mechanical description of physical reality be considered complete?,” Physical Review, vol. 48, no. 8, pp. 696-702, Oct (1935)

[4] E. Schrodinger, “The current situation in quantum mechanics,” Naturwissenschaften, vol. 23, pp. 807-812, (1935)

[5] W Heisenberg, Physics and beyond : Encounters and conversations (Harper, New York, 1971)